Statistics for Decision Makers - 05.06 - Probability - Poisson Distribution
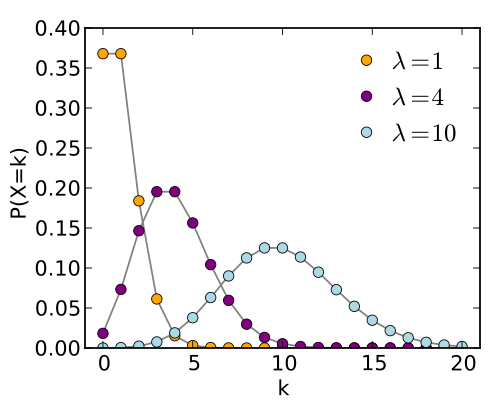
Poisson Distribution。
Calculating randomly scattered events in time or in space.
- Examples
- The number of road accidents in given period.
- The goals scored in a soccer match.
- The number of Losses/Claims occurring in a given period.
- The number of customers calling in a day.
Formula。
In order to apply the Poisson distribution, the various events must be independent.
The general formula of the Poisson distribution is:
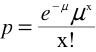e is the base of natural logarithms (2.7183)
μ is the mean number of "successes"
x is the number of "successes" in question
Example。
- The mean number of customer calls to your company on a weekday is 8.
- What is the probability that on a given weekday there would be 11 calls?
- Solution
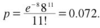In a spreadsheet
=POISSON(11,8,false)
The probability of having 11 calls is 0.072.
Quiz。
Quiz
<quiz display=simple>
{The mean number of defective products produced in a factory in one day is 21. What is the probability that in a given day there are exactly 12 defective products?
|type="{}"} { 0.012 | .012 }
{
Answer >>
0.012
0.012 can be obtained using the formula.
}
{Which of these can be computed using the Poisson distribution?
|type="[]"}
-The average waiting time between phone calls.
+The number of people killed accidentally by horse kicks.
{Which of these can be computed using Poisson distribution? |type="[]"} +The number of enquiries via online form in a month. -The probability of selecting a person over 2 metres high.
