Areas of Normal Distributions
Jump to navigation
Jump to search
- Areas under portions of a normal distribution can be computed by using calculus.
- Since this is a non-mathematical treatment of statistics, we will rely on computer programs and tables to determine these areas.
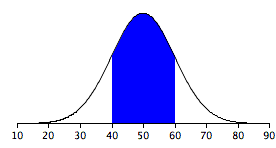
Example 1
It shows a normal distribution with
- a mean of 50
- a standard deviation of 10
The shaded area between 40 and 60 contains 68% of the distribution.
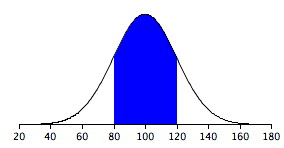
Example 2
It shows a normal distribution with
- a mean of 100
- a standard deviation of 20.
As in Example 1, 68% of the distribution is within one standard deviation of the mean.
The normal distributions shown in Example1 and 2 are specific examples of the general rule that 68% of the area of any normal distribution is within one standard deviation of the mean.
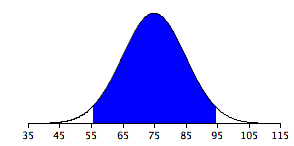
Example 3
It shows a normal distribution with
- a mean of 75
- a standard deviation of 10
The shaded area contains 95% of the area and extends from 55.4 to 94.6.
95% of the Area
- For all normal distributions, 95% of the area is within 1.96 standard deviations of the mean.
- For quick approximations, it is sometimes useful to round off and use 2 rather than 1.96 as the number of standard deviations you need to extend from the mean so as to include 95% of the area.
Quiz